Continuity and Differentiability Algebra and Calculu

1. Limits –

For a function $f(x)$ the limit of the function at a point $x=a$ is the value the function achieves at a point which is very close to $x=a$ .

Formally,
Let $f(x)$ be a function defined over some interval containing $x=a$ , except that it
may not be defined at that point.
We say that, $L = \lim_{x\to a} f(x)$ if there is a number $\delta$ for every number $\epsilon$ such that
$|f(x)-L| < \epsilon$ whenever $0<|x-a|<\delta$

The concept of limit is explained graphically in the following image –
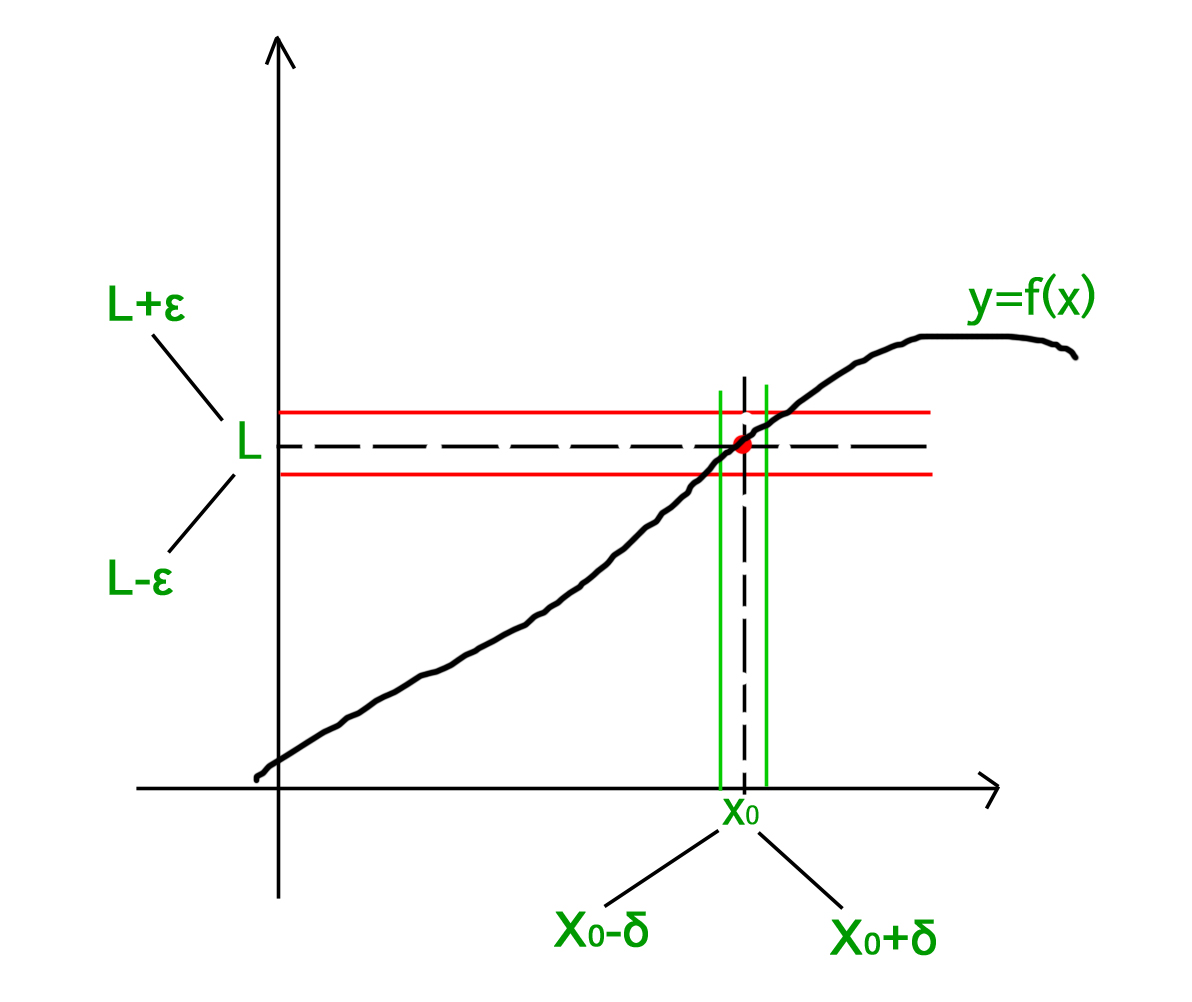
Definition of limit graphical
As is clear from the above figure, the limit can be approached from either sides of the number line i.e. the limit can be defined in terms of a number less that $a$ or in terms of a number greater than $a$ . Using this criteria there are two types of limits –
Left Hand Limit – If the limit is defined in terms of a number which is less than $a$ then the limit is said to be the left hand limit. It is denoted as $x\to a^-$ which is equivalent to $x=a-h$ where $h>0$ and $h\to 0$ .
Right Hand Limit – If the limit is defined in terms of a number which is greater than $a$ then the limit is said to be the right hand limit. It is denoted as $x\to a^+$ which is equivalent to $x=a+h$ where $h>0$ and $h\to 0$ .

Existence of Limit – The limit of a function $f(x)$ at $x=a$ exists only when its left hand limit and right hand limit exist and are equal and have a finite value i.e.
$\lim_{x\to a^-}f(x) = \lim_{x\to a^+}f(x)$

Some Common Limits –

$\begin{align*} &\bullet\: \lim_{x\to 0} \frac{\sin x}{x} = 1 \hspace{0.5cm}& &\bullet\: \lim_{x\to 0} \cos x = 1 \hspace{0.5cm}& &\bullet\: \lim_{x\to 0} \frac{\tan x}{x} = 1& \\ &\bullet\: \lim_{x\to 0} \frac{1-\cos x}{x} = 0 \hspace{0.5cm}& &\bullet\: \lim_{x\to 0} \frac{\sin x^\circ}{x} = \frac{\pi}{180} \hspace{0.5cm}& &\bullet\: \lim_{x\to a} \frac{x^n - a^n}{x-a} = na^{n-1}& \\ &\bullet\: \lim_{x\to \infty} (1+\frac{k}{x})^{mx} = e^{mk} \hspace{0.5cm}& &\bullet\: \lim_{x\to 0} (1+x)^\frac{1}{x} = e \hspace{0.5cm}& &\bullet\: \lim_{x\to 0} \frac{(a^x-1)}{x} = \ln {a} \hspace{0.5cm}& \\ &\bullet\: \lim_{x\to 0} \frac{e^x-1}{x} = 1 \hspace{0.5cm}& &\bullet\: \lim_{x\to 0} \frac{\ln {(1+x)}}{x} = 1 \hspace{0.5cm}& &\bullet\: \lim_{x\to \infty} x^\frac{1}{x} = 1 \hspace{0.5cm}& \\ \end{align}$

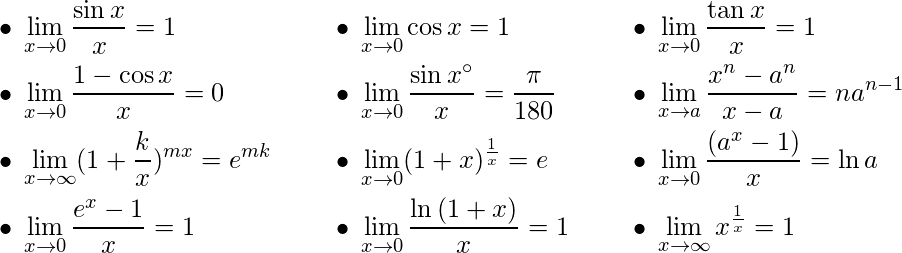
L'Hospital Rule –
If the given limit $\lim_{x\to a} \frac{f(x)}{g(x)}$ is of the form $\frac{0}{0}$ or $\frac{\infty}{\infty}$ i.e. both $f(x)$ and $g(x)$ are 0 or both $f(x)$ and $g(x)$ are $\infty$ , then the limit can be solved by L'Hospital Rule.
If the limit is of the form described above, then the L'Hospital Rule says that –
$\lim_{x\to a}\frac{f(x)}{g(x)} = \lim_{x\to a}\frac{f^\prime(x)}{g^\prime(x)}$
where $f^\prime(x)$ and $g^\prime(x)$ obtained by differentiating $f(x)$ and $g(x)$ .
If after differentiating, the form still exists, then the rule can be applied continuously until the form is changed.

Example 1 – Evaluate $\lim_{x\to 0} \frac{x\cos{(x)}-\sin{(x)}}{x^2\sin{(x)}}$
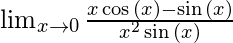
Solution – The limit is of the form 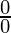$\frac{0}{0}$ , Using L'Hospital Rule and differentiating numerator and denominator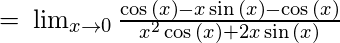
$=\:\lim_{x\to 0} \frac{\cos{(x)}-x\sin{(x)}-\cos{(x)}}{x^2\cos{(x)} + 2x\sin{(x)}}$

$=\:\lim_{x\to 0} \frac{-\sin{(x)}}{x\cos{(x)} + 2\sin{(x)}}$

$=\:\lim_{x\to 0} \frac{\frac{-\sin{(x)}}{x}}{\cos{(x)} + \frac{2\sin{(x)}}{x}}$

$=\:\frac{-1}{1 + 2*1}$

$=\:\frac{-1}{3}$
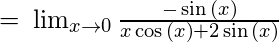
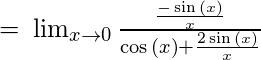
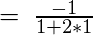
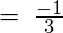
Example 2 – Evaluate $\lim_{x\to 0}\frac{a^{mx}-b^{nx}}{\sin {kx}}$
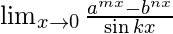
Solution – On multiplying and dividing by 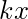$kx$ and re-writing the limit we get –
$=\:\lim_{x\to 0}\frac{(a^{mx}-1) - (b^{nx}-1)}{kx}.\frac{kx}{\sin {kx}}$
$=\:\frac{1}{k}\lim_{x\to 0}(\frac{((a^m)^{x}-1)}{x} - \frac{((b^n)^{x}-1)}{x}).\lim_{x\to 0}\frac{kx}{\sin {kx}}$

$=\:\frac{1}{k}(\ln {a^m} -\ln {b^n}).1$

$=\:\frac{1}{k}\ln {\frac{a^m}{b^n}$

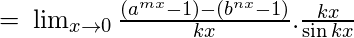
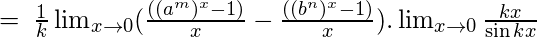
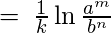
2. Continuity –

A function is said to be continuous over a range if it's graph is a single unbroken curve.
Formally,
A real valued function $f(x)$ is said to be continuous at a point $x=x_\circ$ in the domain if –
$\lim_{x\to x_\circ} f(x)$ exists and is equal to $f(x_\circ)$ .
If a function $f(x)$ is continuous at $x=x_\circ$ then-
$\lim_{x\to x_\circ ^+} f(x) = \lim_{x\to x_\circ ^-} f(x) = \lim_{x\to x_\circ} f(x)$
Functions that are not continuous are said to be discontinuous.

3. Differentiability –

The derivative of a real valued function $f(x)$ wrt $x$ is the function $f^\prime(x)$ and is defined as –
$\lim_{h\to 0} \frac{f(x+h)-f(x)}{h}$

A function is said to be differentiable if the derivative of the function exists at all points of its domain. For checking the differentiability of a function at point $x=c$ ,
$\lim_{h\to 0} \frac{f(c+h)-f(c)}{h}$ must exist.

If a function is differentiable at a point, then it is also continuous at that point.
Note – If a function is continuous at a point does not imply that the function is also differentiable at that point. For example, $f(x) = |x|$ is continuous at $x=0$ but it is not differentiable at that point.

GATE CS Corner Questions

Practicing the following questions will help you test your knowledge. All questions have been asked in GATE in previous years or in GATE Mock Tests. It is highly recommended that you practice them.

1. GATE CS 2013, Question 22
2. GATE CS 2010, Question 5
3. GATE CS 2008, Question 1
4. GATE CS 2007, Question 1
5. GATE CS 2015 Set-1, Question 14
6. GATE CS 2015 Set-3, Question 19
7. GATE CS 2016 Set-1, Question 13
8. GATE CS 1998, Question 4

References-

Continuity – Wikipedia
Limits – Wikipedia
Differentiability – Wikipedia
This article is contributed by Chirag Manwani. If you like GeeksforGeeks and would like to contribute, you can also write an article using contribute.geeksforgeeks.org or mail your article to contribute@geeksforgeeks.org. See your article appearing on the GeeksforGeeks main page and help other Geeks.

Please write comments if you find anything incorrect, or you want to share more information about the topic discussed above.

hesterthumbly.blogspot.com

Source: https://www.geeksforgeeks.org/mathematics-limits-continuity-differentiability/

Continuity and Differentiability Algebra and Calculu

1. Limits –

2. Continuity –

3. Differentiability –

0 Response to "Continuity and Differentiability Algebra and Calculu"

Post a Comment

Iklan Atas Artikel

Iklan Tengah Artikel 1

Iklan Tengah Artikel 2

Iklan Bawah Artikel